Mode-Locked Lasers
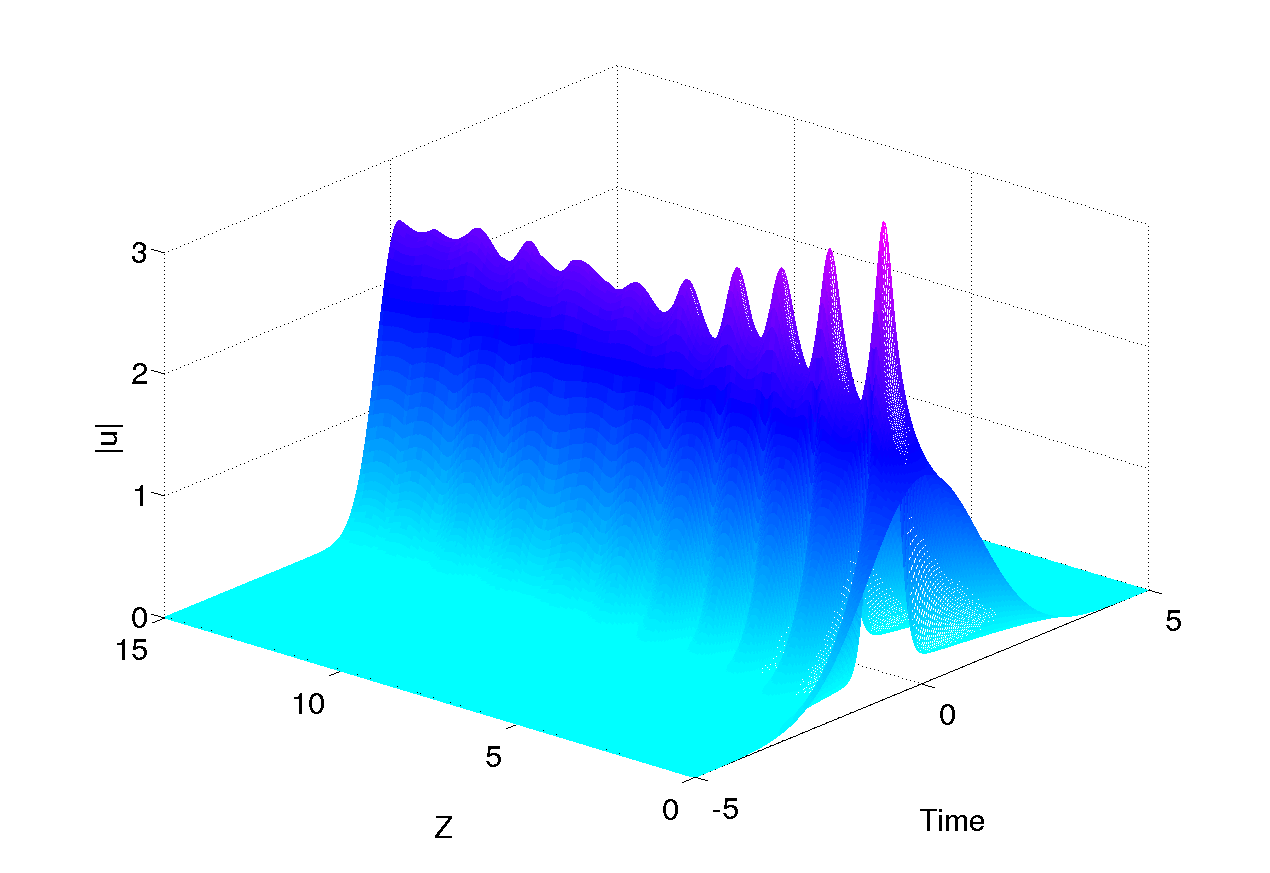
My current primary research interests is in modeling mode-locked lasers. Mode-locked lasers are capable of producing a continuous stream of pulses with extremely high peak power and ultrashort duration, two properties that have greatly advanced the fields of extreme nonlinear optics, optical frequency metrology, and laser spectroscopy, among others. Most modern MLLs are passively mode-locked through a combination of a nonlinear gain and saturable loss, the latter of which is usually achieved through semiconductor saturable absorbers or, more recently, by coupling the cavity to an external waveguide array.
The prevailing efforts to predict the performance of mode-locked lasers are largely based on models of pulses propagating in optical communication systems. While the pulse dynamics on intermediate time or length scales, e.g. those due to dispersion and nonlinearity, is very similar between the two cases, there exist other components of mode-locked lasers that operate on much longer length scales. For example, the saturating absorption responsible for pulse formation typically has a response time that is much longer than the transit time of a pulse through the laser cavity, even though it is often modeled as occurring instantaneously. In addition, the electronic feedback that controls the pulse timing and phase operates on an even longer time scale. We are currently attempting to develop a more self-consistent model, starting from Maxwell's equations, that simultaneously includes the constitutive gain/loss dynamics and electronic feedback with which the pulse evolution is coupled.
Rare Events in Lightwave Systems
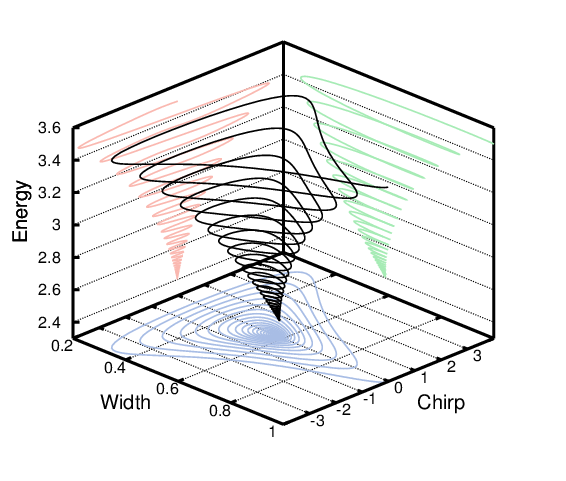
MLLs are very stable devices, which is one reason why they are so useful. Because they are so stable, quantifying their performance in the presence of noise presents a difficult challenge. Of primarily interest is the likelihood of the laser producing unusable output, which is an extremely rare event by design and therefore ill-suited to conventional Monte Carlo approaches. One solution to this problem is to use variance reduction techniques such as importance sampling in conjunction with a Monte Carlo method to sample these rare events more frequently. The difficulty is then finding the most probable noise realizations, or paths, that lead to failures in the system. Not only are these realizations not obvious a priori, but different failure mechanisms might become relevant at different parameter regimes and different system tolerances.
Viewed mathematically, the stability of MLLs is attributed to the existence of a basin of attraction to a fixed point that physically corresponds to a stable mode of operation. If noise present is present in the system, the pulse will fluctuate randomly about this fixed point, and on rare occurrences, the noise will produce trajectories that escape from the basin of attraction, corresponding physically to a failure event. Mathematically, this is referred to as an exit problem, which is concerned with calculating quantities such as the probability of an exit occurring and mean exit time, both of which can, in theory, be addressed through the methods of computational probability and Monte Carlo methods.